






Differential Equations Steps

Deskripsi Differential Equations Steps
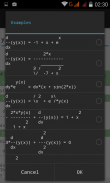
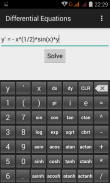
Generally, differential equations calculator provides detailed solution
Online differential equations calculator allows you to solve:
Including detailed solutions for:
[✔] First-order differential equations
[✔] Linear homogeneous and inhomogeneous first and second order equations
[✔] A equations with separable variables
Examples of solvable differential equations:
[✔] Simple first-order differential equations
[✔] Differential equations with separable variables
[✔] Inhomogeneous linear differential equation of first order
[✔] Bernoulli differential equation
[✔] Exact differential equations
[✔] Linear homogeneous second order differential equations with constant coefficients
[✔] Inhomogeneous linear second order differential equations with constant coefficients
[✔] Differential equations, allowing reduction of the order
[✔] Linear homogeneous and inhomogeneous differential equations of higher order with constant coefficients
[✔] Supported all math symbols and functions. For example: sin(x), cos(x), exp(x), tan(x), ctan(x) and other.
[✔] Suported complex variables (solve complex equations)
The Calculator contain several features:
[✔] Several examples
[✔] Сorrect input expression errors
Examples for solve:
y' = x + e^x - 1
y' = 2x/(x^2 - 7)^(1/3)
e^y*dy = (x + sin(2x))dx
y' = y*(x^2 + e^x)
y' - 2*x*y/(1+x^2) = 1 + x^2
y'' + 3*y' = 0
x*y'' - xy' + y = 0
y'' - 2y' = (x^2 + 1)*e^x
x*y'' - xy' + y = x^2 + 1
(x^2 - y^2)dx - 2xydy = 0
y'''' + y''' - 5y'' + y' - 6y = x*cos(x) + sin(x)
4y^3*y'' = y^4 - 1</br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br></br>





























